I share here the slides of the keynote presentation I prepared for the 9th International Conference on Spectroscopic Ellipsometry ICSE-9.
Title: Exploring the sensitivity limits of a Mueller matrix ellipsometer. The spatial dispersion of Si

I share here the slides of the keynote presentation I prepared for the 9th International Conference on Spectroscopic Ellipsometry ICSE-9.
Title: Exploring the sensitivity limits of a Mueller matrix ellipsometer. The spatial dispersion of Si
I have been appointed as Topical Editor in Applied Optics in the areas of Polarization, Polarimetry, Ellipsometry, and Polarimetric Imaging. Applied Optics, from Optica Publishing Group (formerly Optical Society of America), is my top preferred journal for applications-centered research in optics, and I have published there many articles. I hope I will receive many interesting submissions related to these topics and, therefore, I also encourage researchers working on polarization, polarimetry and ellipsometry to consider Applied Optics as a suitable journal for their best works.
The purpose of this post is to provide some interesting documents related to the history of ellipsometry. It is sometimes considered that Paul Drude was the “inventor” of ellipsometry. Despite the many contributions of P. Drude, in my opinion this statement is not true and, as almost all aspects and techniques related with the polarization of light, ellipsometry was invented in France, as early as mid XIXth century. It is however true, that at that time the influence of thin films on ellipsometry measurements was still not discovered or understood (althought the associated ellipticity was already measured), so the technique was mostly regarded as a method to determine optical constants of bulk materials. The relation between ellipsometry and thin films came later, with the works of P. Drude and Lord Rayleight.
Jules Célestin Jamin (1818-1886). If ellipsometry needs an inventor, it is him. He did many ellipsometry measurements and described the first ellipsometer, which was later made commercial by French optic intrument makers Soleil/Duboscq.
Here I share two of his publications from 1847 and 1850, which clearly describe how the polarization ellipse is measured upon light reflection on different materials.
Samuel Haughton (1821-1897) made a great English summary of Jamin’s Publications and he himself made many more measurements using an ellipsometer that replicated Jamin’s description.
Jamin’s 1850 publication gives a detailed description of the instrument he was using. This instrument was made commercial by the French Scientific Instrument Makers Soleil, Duboscq, and their successors. The instrument was sold under the name of “Grand cercle de Jamin et Sernármont” and comprised one rotatable polarizer, one rotatable analyzer and an ajustable compensator. The “grand cercle” was used to adjust the angle of incidence. It seems it was possible to purchase this instrument during 1870s, possibly even earlier.

The image capture above comes from this catalog of instruments:
Interestingly, the instrument could be used both horizontally or vertically, allowing the sample to sit horizantally when needed (this was for example essential for the measurement of the refractive angle of liquids, which they did). I have found this photo of the grand “cercle” mounted vertically (samples horizontal then), although I am afraid that whoever took this photo did not arrange “ellipsometer arms” in a reasonable configuration.

I am looking for PhD cantidates to be funded by the 2022 FI scholarship program from Catalan research Agency. Students with a good academic record interested in developing a PhD in Physical Optics with a focus on the polarization of light can contact me,
More info:
Applications opened only until October 20! These are competitive positions but candidates with a good CV have a high success rate.
Local students are especially welcomed to apply.
FI scholarships offer good economic conditions and they also cover academic fees, other training activities and research stays.
We are curently working on an upgraded Mueller matrix microscope allowing for fast MM imaging. Stay tuned for more information about it!
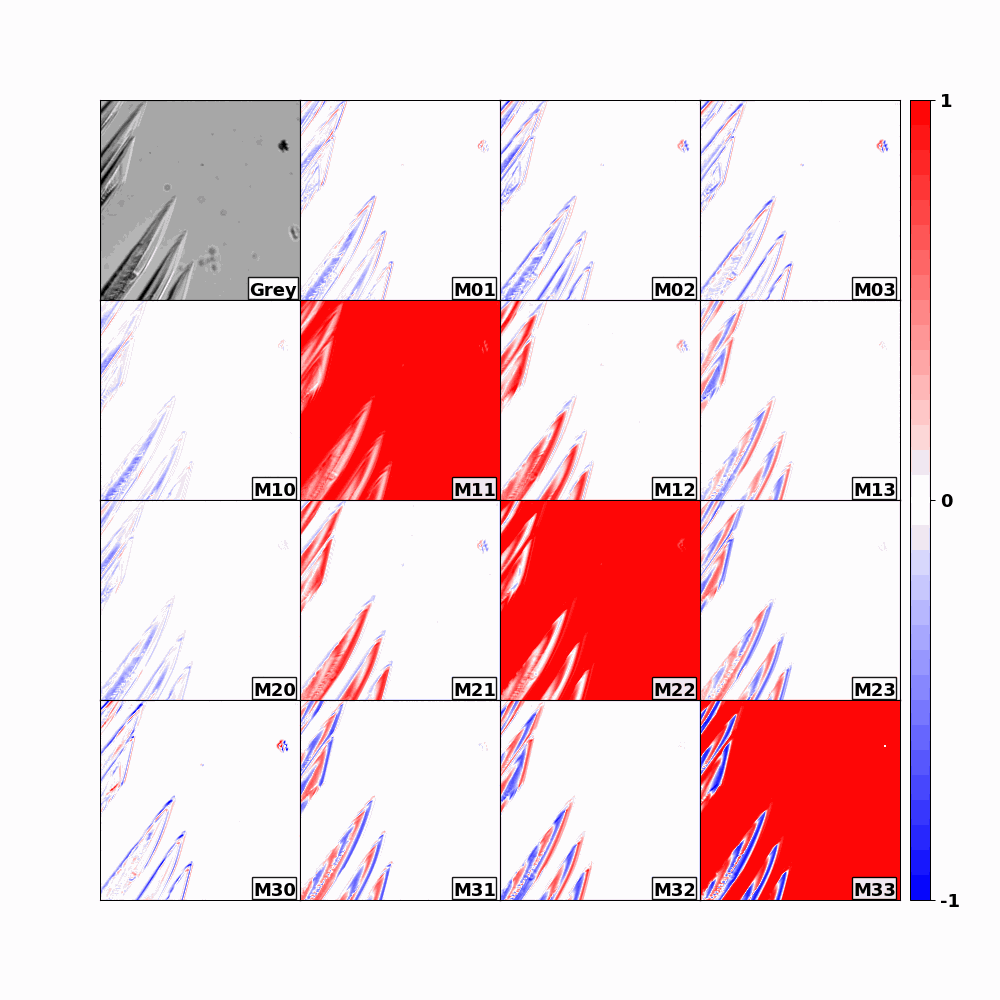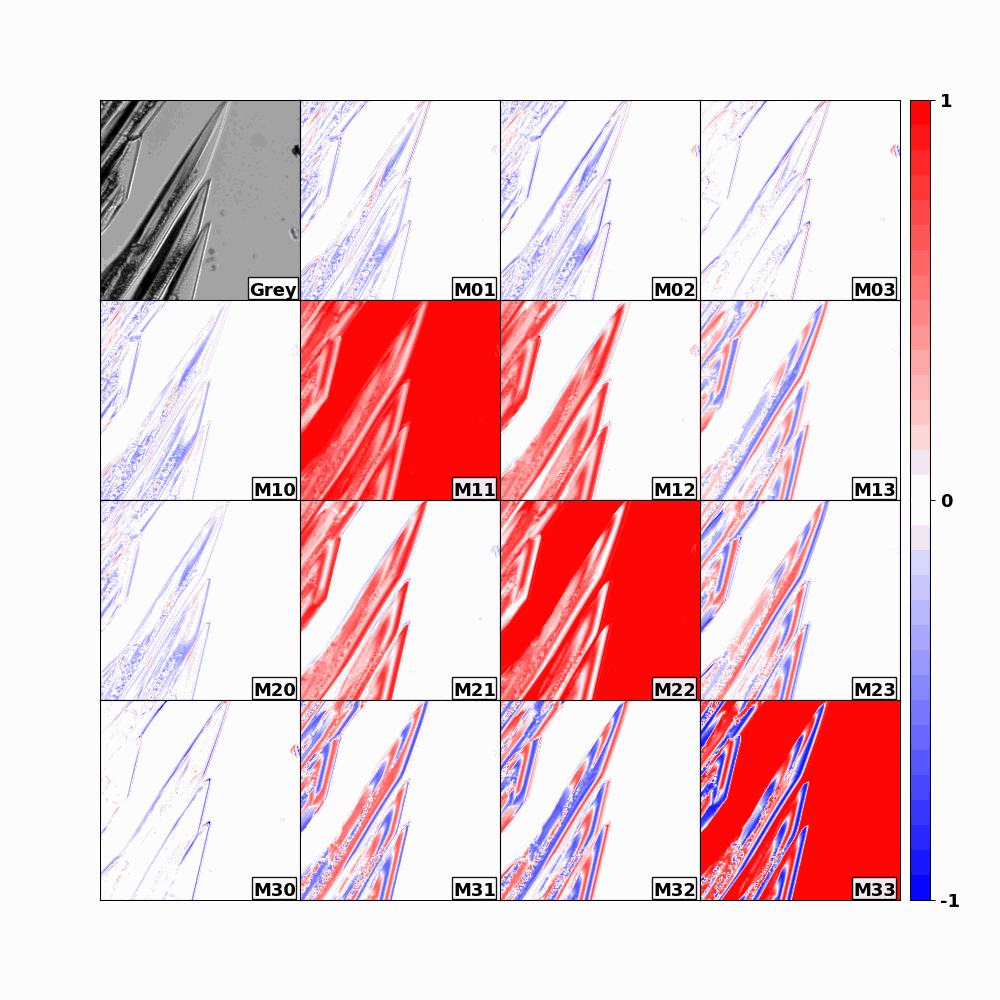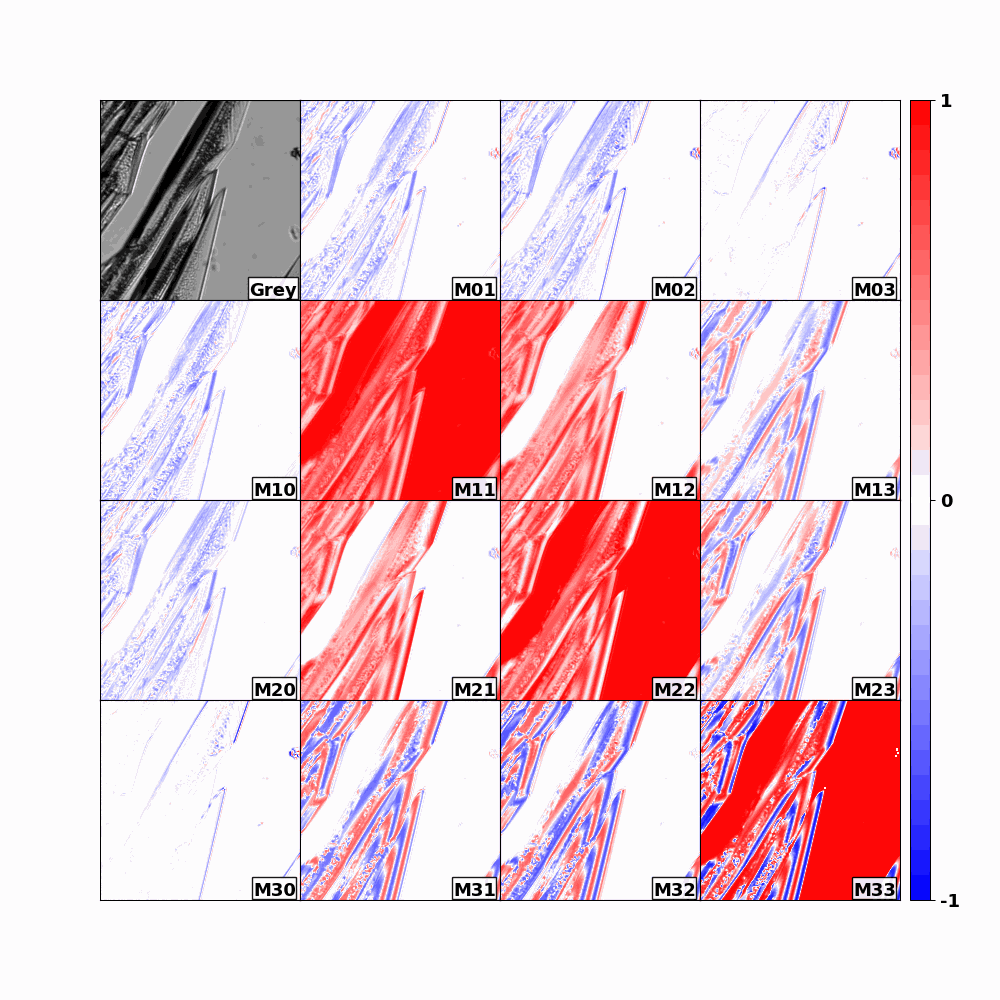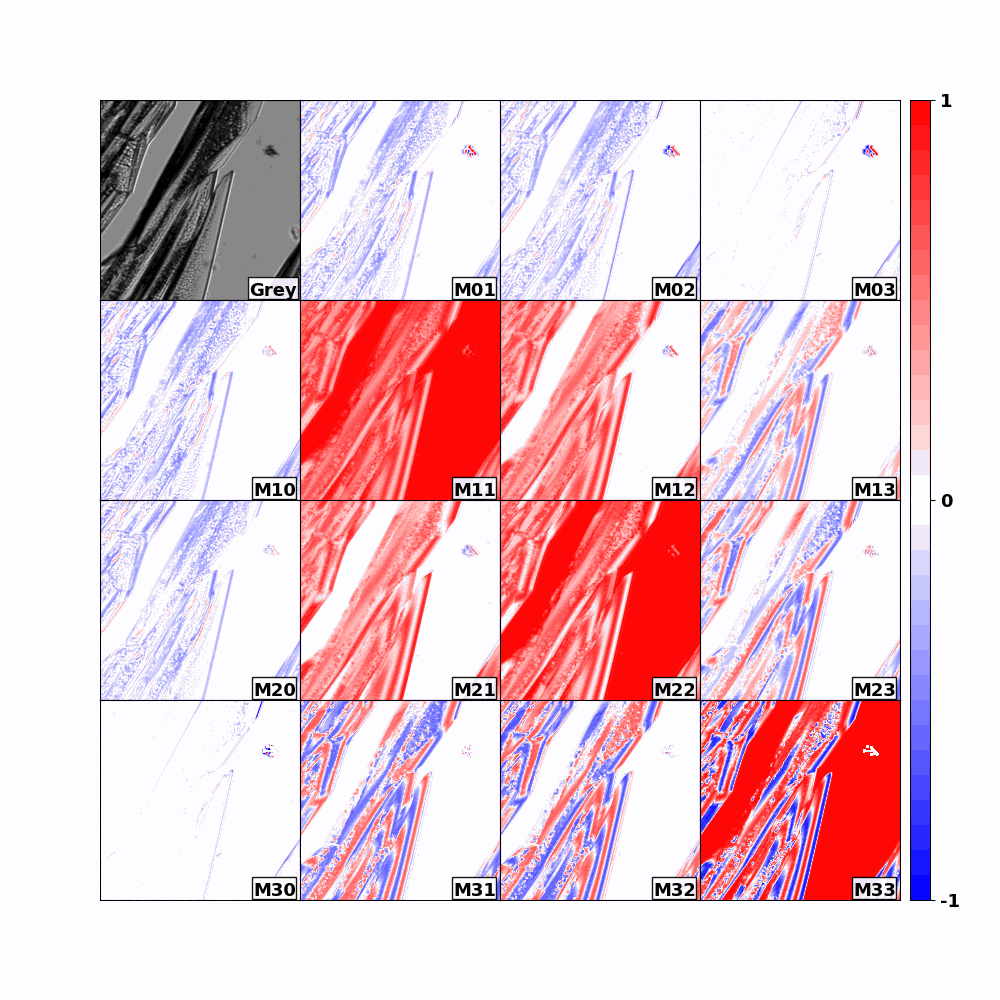
Animation of growing Nickel sulphate crystals by Europhotonics Master Student Dale Gottlieb
This ellipsometer was build in our Lab during 2019 and 2020 in joint work with visiting student Subiao Bian (Huaqiao University, China). Has several features: compensation using Fresnel Rhombs and discrete angle positioning that makes it an interesting device. The manuscript has been selected for an Editor’s Pick in Applied Optics.
https://www.osapublishing.org/ao/abstract.cfm?uri=ao-60-16-4964
I have recently published a new paper in J. Mod. Opt. about something I discovered when reading Fresnel’s Oeuvres Complètes and that relates to the geometric phase!
https://doi.org/10.1080/09500340.2021.1898687

I am sharing here the slides of a presentation I did for the course Séminaires en sciences physiques, from Université de Moncton, in Quebec, Canada.
Phase and polarization are the two main actors of this presentation. The ‘phase’ describes the cyclic change of electric and magnetic fields during one period of the wave, while the ‘polarization’ describes the shape and orientation of the path traced by the electric field during one period. This presentation will look back into two historical experiments that made revolutionary steps for understanding the interference of polarized beams of light, but that are often omitted in textbooks of current use. The first experiment is from the early XIXth century and served Fresnel and Arago to define their laws of interference. The second experiment is from mid XXth century when S. Pancharatnam pioneered the unintuitive concept of geometric phase to explain the results of his apparently simple interference experiments in crystals. Finally, we will study the ever-lasting influence of these experiments in modern research, especially in the novel designed metasurfaces for photonics applications.
I got involved in the committe of this new webinar series that has been promoted by Thomas Germer.
This series of webinars will bring together an international audience interested in exploring topics associated with optical polarization and related phenomena. Monthly webinars will be presented by leaders in the field, and ample time will be provided for live discussion of the topic. Initiated during the COVID-19 pandemic, the series will attempt to replicate as much as possible the dynamics of in-person conferences.
More information is available https://groups.google.com/g/the-henri-poincare-webinar-series and you can join there the mailing list.
REGISTER: HERE
The scheduled initial speakers are:
• September 29, 2020 – 1500 UTC – Miguel Alonzo (Institut Fresnel)
• October 27, 2020 – 1300 UTC – Federico Capasso (Harvard University)
• November 24, 2020 – 1300 UTC – Frans Snik (Universiteit Leiden)
• December 29, 2020 – open
• January 26, 2020 – 1300 UTC – Bart Kahr (New York University)
Henry Poincaré did many things in Polarization Optics but AFAIK he never draw his famous Sphere.
I have mixed feeling about journal reviewing. I generally like it, but it certainly takes a lot of time and it is not always the case that you feel that this time is truly profitable. So I think it is good that at least journals make some sort of recogniztion to reviewers. This year I have received from OSA the Outstanding Reviewer Recognition. The OSA Outstanding Reviewer recognition is given annually to commend the top reviewers for their outstanding peer review efforts over the past year.